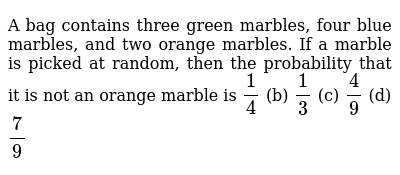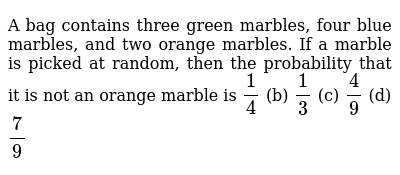A Bag Contains 6 Red Marbles 2 Blue Marbles And 1 Green Marble Find P Not Blue

A marble is selected at random from the bag and.

A bag contains 6 red marbles 2 blue marbles and 1 green marble find p not blue.

A bag contains 4 yellow 2 red and 6 green marbles. A bag of marbles contains six red marbles four blue marbles eight green marbles and two yellow marbles. Red yellow and blue a jar contains 30 red marbles 12 yellow marbles 8 green marbles and 5 blue marbles you draw and replace marbles 3 times. Two consecutive draws are made from the bag without replacement of the first draw.

The probability of picking a blue one is 4 20. Calculate the expected value of the random variable. Answer by richwmiller. In order to find the probability of picking a yellow marble from a bag we have to first determine the number of possible outcomes and then how many of them meet our constraints.

A bag contains 2 red marbles 3 blue marbles and 7 green marbles. Two 6 sided dice are rolled. The first is replaced before the second is drawn. What is the probability you draw 1 red 1 yellow and 1 blue.

If a marble is selected at random what is the probability that is is not blue. If a marble is randomly selected from the bag what is the probability that it is blue. This is harder because we are drawing marbles in an order but we don t care about which order we. A bin contains 72 light bulbs of which 10 are defective.

What is the probability that the sum of the two numbers on the dice will be 3. If 5 light bulbs. If a marble is selected at random what is the probability that is is not blue. A bag contains 5 red marbles 4 blue marbles and 1 green marble.

A random variable assigns the number of red marbles to each outcome. Express the indicated degree of likelihood as a probability value. A bag contains 3 red marbles 5 green marbles and 2 blue marbles. 6 18 33 3.

It will turn dark tonight after the sun sets completeley. 8 6 4 18. 20 marbles are in the bag four of them are blue. Two marbles are drawn.

Remember that first you need to select the red marble 33 3 then select the green marble 22 2.